Abstract :
We investigate wave propagation in a heterogeneous medium. A
disturbance (the deformation in an elastic wave or the magnetic field in
an electro-magnetic wave) is described by a second order wave equation
in divergence form,
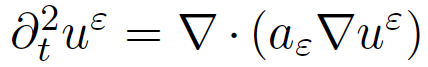
We assume that the coefficient is of the form
![]()
such that the medium has heterogeneities on the small scale "epsilon".
According to classical homogenization results from the 1990’ies, on time
scales of order 1, the effective properties of the medium are
well-described by the effective wave equation
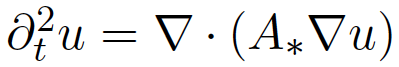
However, when
![]()
dispersive effects have to be taken into account. We demonstrate an analysis of the problem and derive a dispersive effective equation that describes u^epsilon on large time scales.
Numerical tests show the effectiveness of the limit system in one- and two-dimensional examples.
This is joint work with G. Allaire, T. Dohnal, J. Rauch and B. Schweizer.


