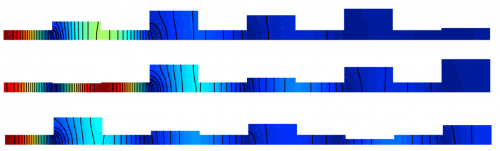
The hallmark of topological phases in periodic materials is the existence of localized modes
on the edges, in addition to bulk waves predicted by the Bloch-Floquet theory. I will discuss
the case of the Su-Schrieffer-Heeger model and its two-dimensional extension. These models
can be exactly realised in acoustic using networks of waveguides. In the one-dimensional
case, edge modes are found and localized near the ends of the system. In two dimensions,
we will analyse the presence of edge waves propagating along a side of the system, but also
modes localized in corners (higher order topological insulator). A key properties of these
topological modes is their robustness to the presence of disorder. We will show theoretically
and experimentally under what precise conditions their existence and eigenfrequency are
maintained when introducing disorder.
LMA - Laboratoire de Mécanique et d’Acoustique
A. Coutant - Topologically protected modes in acoustic networks
Amphithéâtre François Canac, LMA
Le 18 janvier 2022 de 11h00 à 12h00
Antonin Coutant, CR LMA nouvel entrant
Voir en ligne : plus d’informations concernant l’orateur
